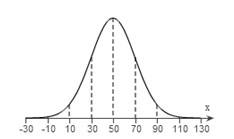
The graph of a normal curve is given. Use the graph to identify the value of µ and σ.
Solution:-
The mean of the distribution µ is the point where the curve reaches the maximum value.
Determine the mean of the graph.
µ = 50
The points at x = µ – σ and x = µ + σ are the inflection points on the normal curve. The inflection points are the points on the curve where the curvature of the graph changes.
The curvature of the accompanying graph change at points x = 30 and x = 70.
Consider the equation for the right inflection point, x = µ + σ.
µ + σ = x
µ + σ – µ = x – µ
σ = x – µ
Find σ.
σ = x – µ
= 70 – 20
Therefore , the mean of the distribution is 50 and the standard deviation is 20.