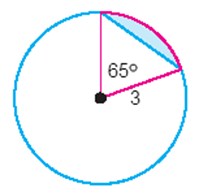
Find the area of the segment of a circle whose radius is 3 feet, formed by a central angle of 65°.
Solution:-
Start by finding the area of the sector formed by a central angle 65°. The area of the sector by multiplying the area of the circle by the ratio of the central angle to 360°.
Area of Sector = ![]()
=![]()
![]() 5.1051
5.1051
The area of the sector is 5.1051 square feet.
Next find the area of the triangle. We know the lengths of two sides of the triangle, and the include angle, so use the formula
K = ![]() a*b*sin
a*b*sin![]()
= ![]() * 3 * 3 sin 65°
* 3 * 3 sin 65°
![]() 4.0784
4.0784
The area of the triangle is 4.0784 square feet.
Subtract the area of the triangle from the area of the sector to find the area of the segment.
Area of segment = 5.1051 – 4.0784
![]() 1.03
1.03
The area of the segment is 1.03 square feet.