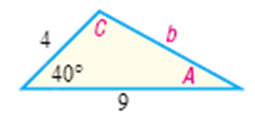
Find the area k of the triangle.
Solution:-
To find the area of the triangle, use the following theorem.
The area K of a triangle equals one-half the product of two of its sides times the sign of their included angle.
According to this theorem, the formula K = 
ac sin B can be used to find the area of the triangle specified in the problem statement.
Using the formula K = ac sin B with a = 4, c = 9, B = 40° given the following result.
K = ac sin B
= * 4 * 9 sin 40°
Simplify the right side to find the area of the triangle.
K 
11.57 square units.